As surely as 2+2=4, it also equals a beginning step on students’ mathematics journey.
Single-digit addition facts like these are so heavily used in mathematics that it’s critical that students ultimately commit them to memory so they don’t have to compute each time they appear.

Research indicates that most students use a variety of increasingly sophisticated strategies as they learn their math facts, and that these strategies ultimately help them store the sums in their long-term memory so they can instantly recall them later. (Some research, in fact, indicates that adults continue to use these strategies long after they’ve become fluent in addition facts—though generally to a lesser degree.)
Take a look at how these strategies develop.
Then, check out our report on math foundations, where you’ll find an explainer and bibliography on math facts, and another aimed at helping teachers and curriculum planners craft a strong approach to fact fluency.
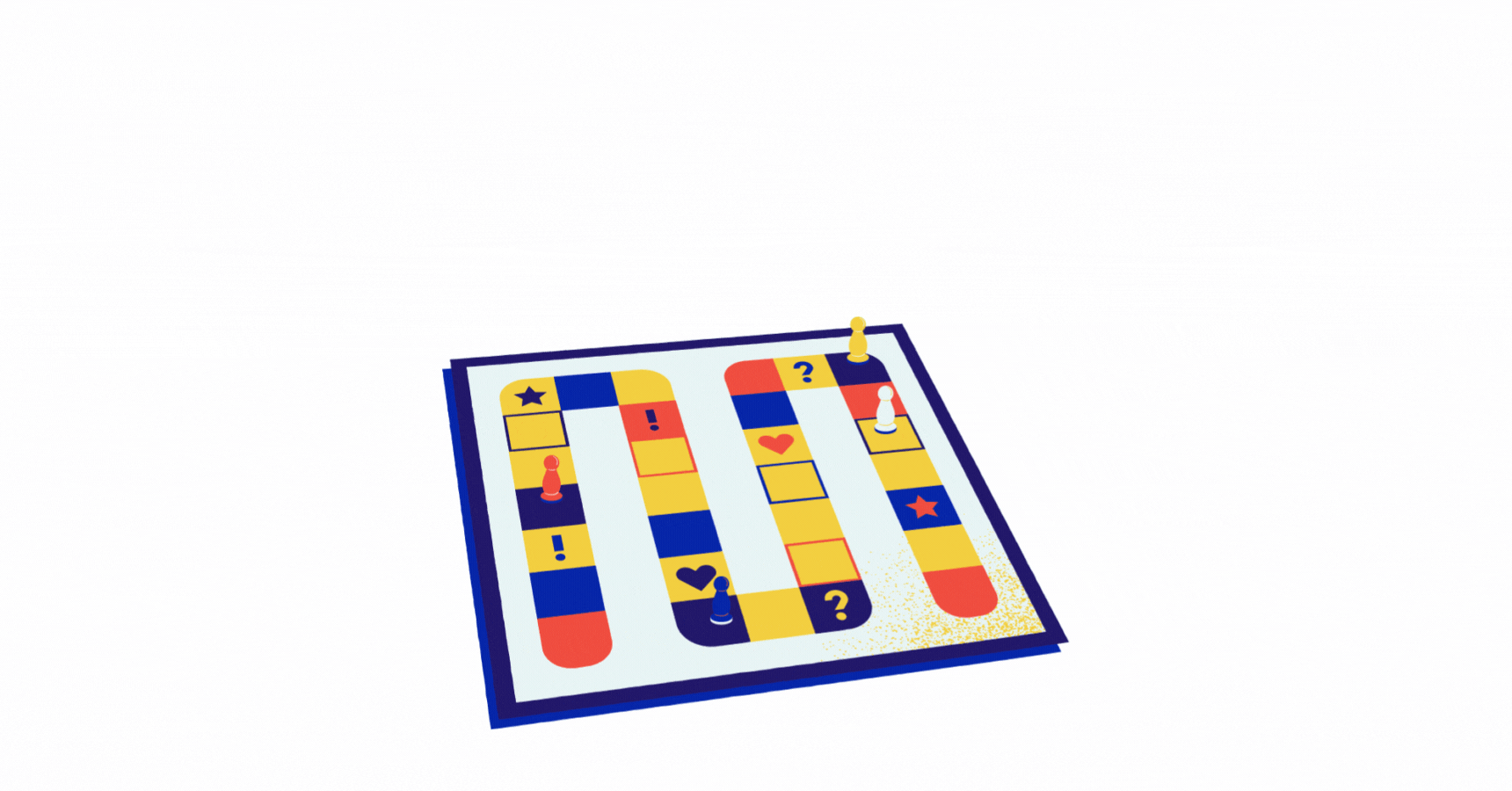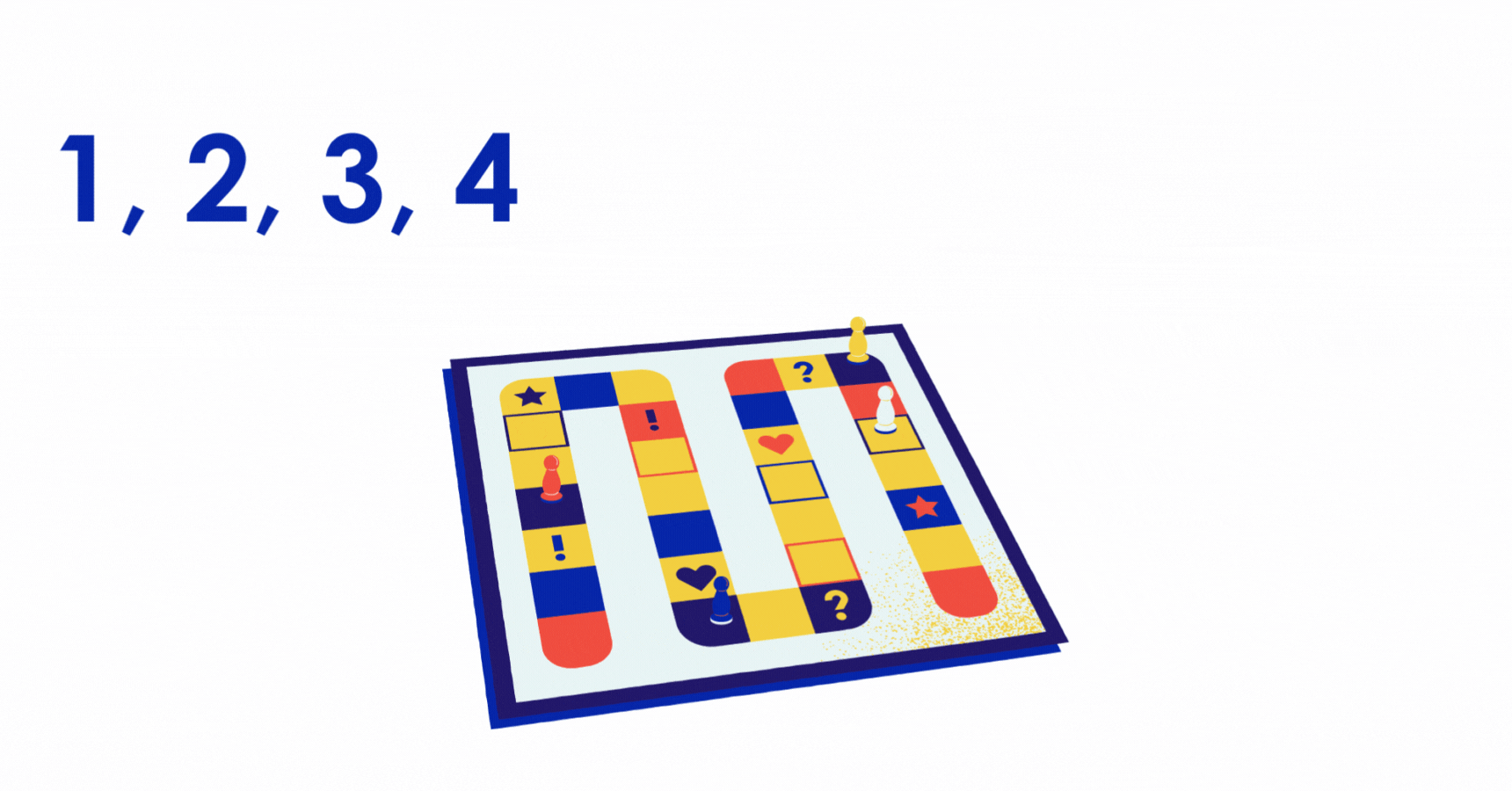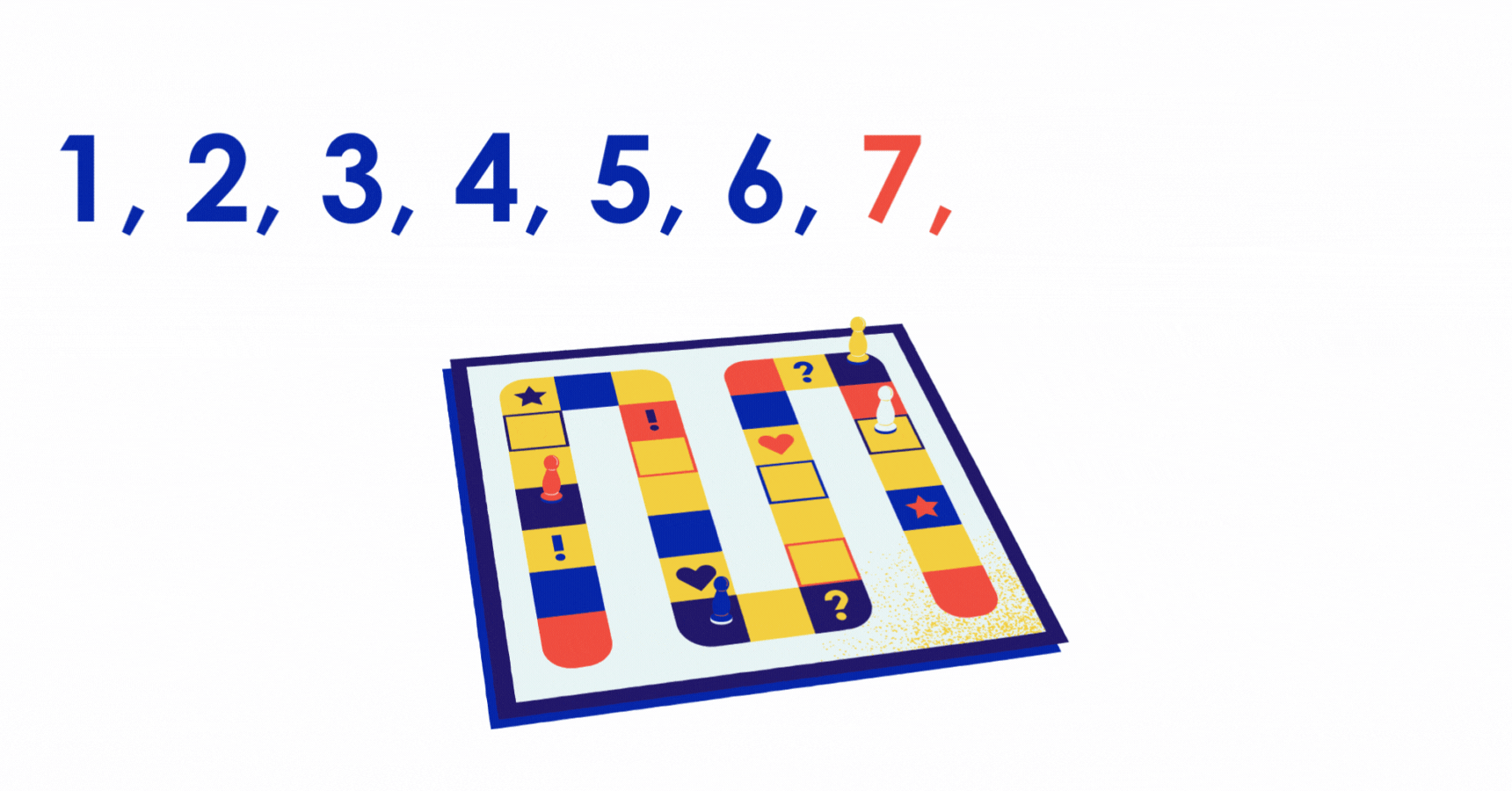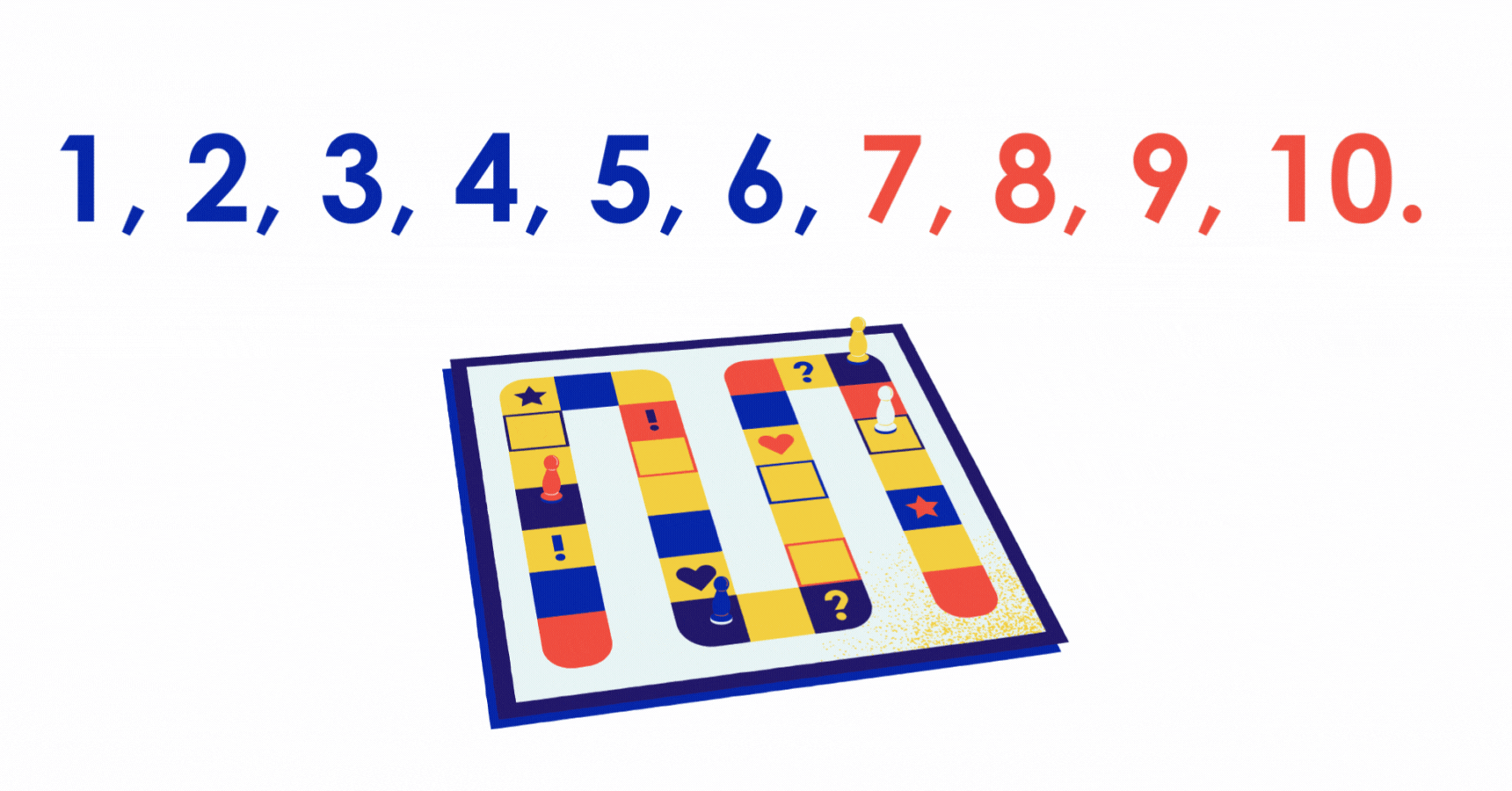
COUNTING ALL
Young students first learn to add by counting every number up to the sum.
COUNTING ON (OR COUNTING UP)
Students realize that the sum is six more than 4 in the counting sequence, and they can simply begin counting from 4.
Eventually, no matter the order of the numbers to be added, or “addends,” they automatically choose the larger and count from there.

�ٷ��䰿�ѱ�O�����ձ�����
Students see that a number can be decomposed into various parts and use that knowledge to make addition easier.
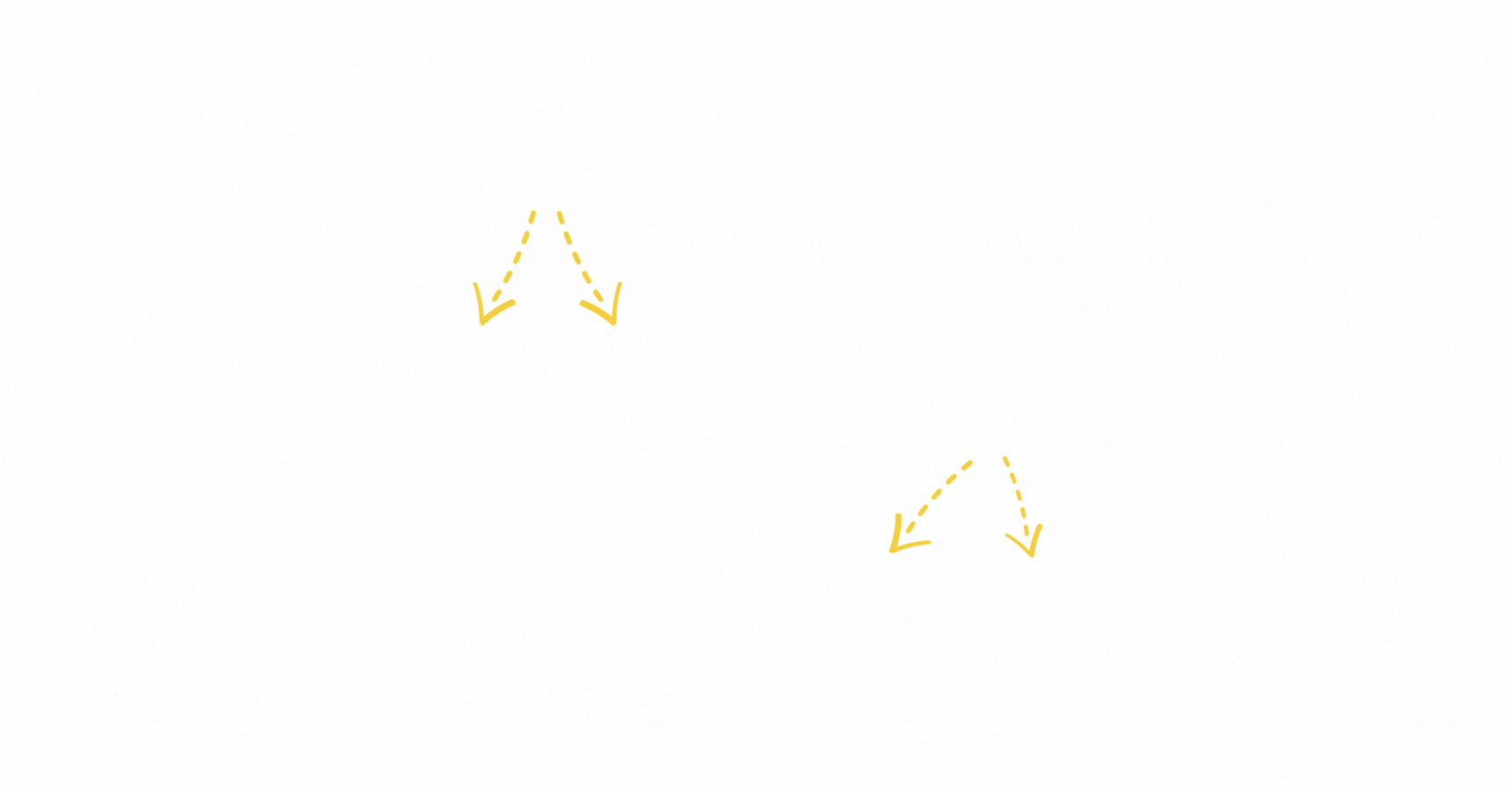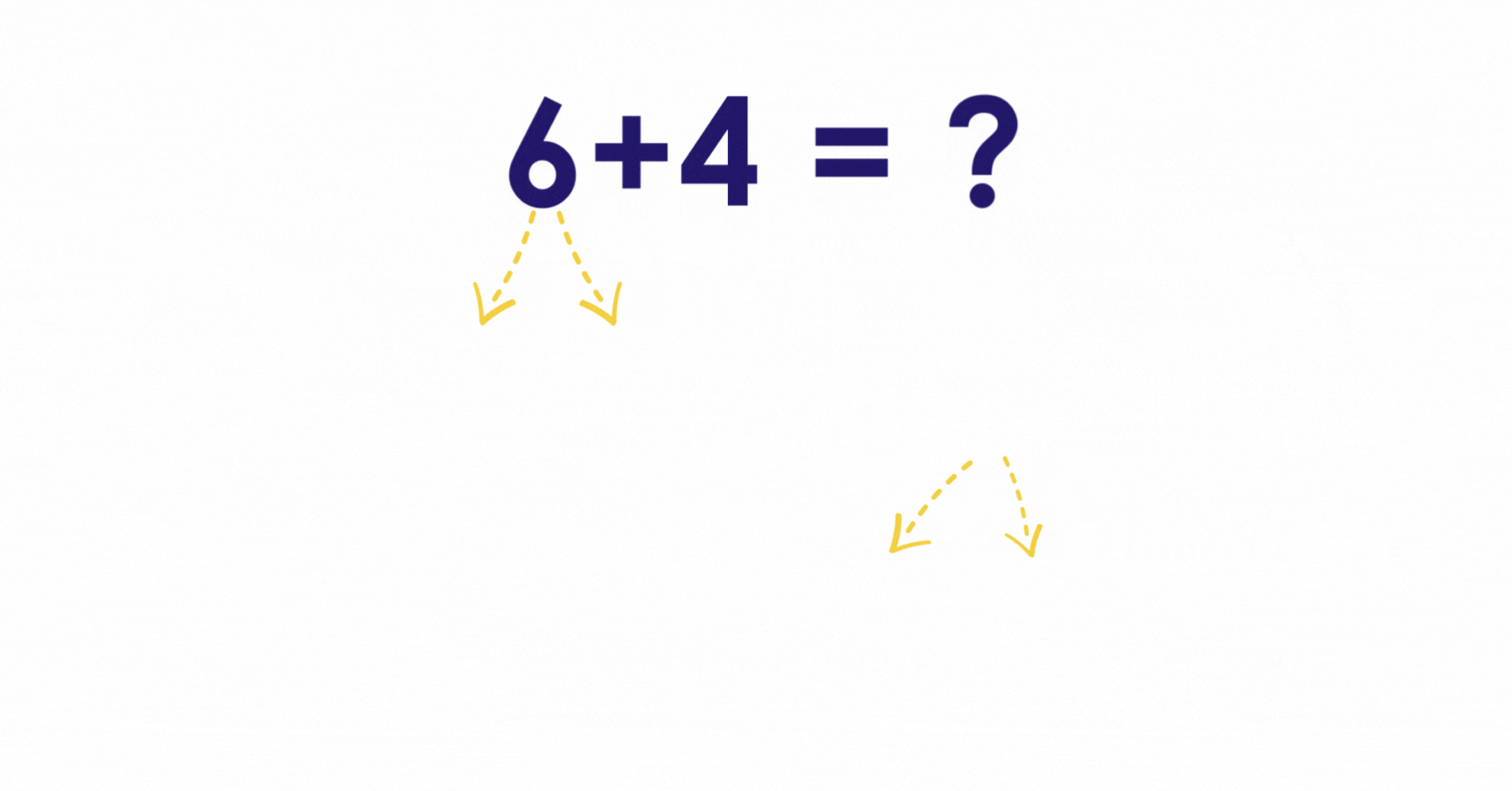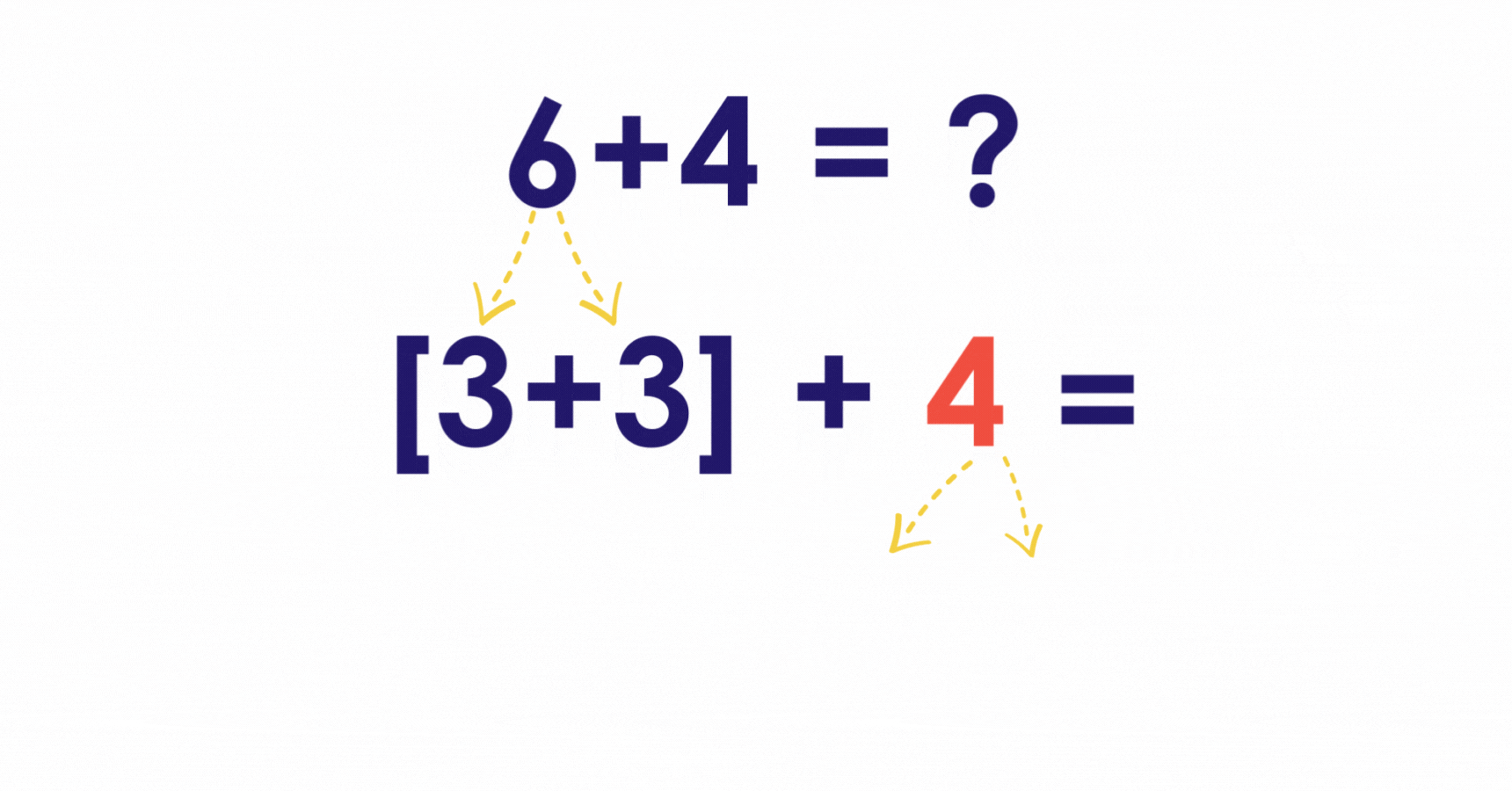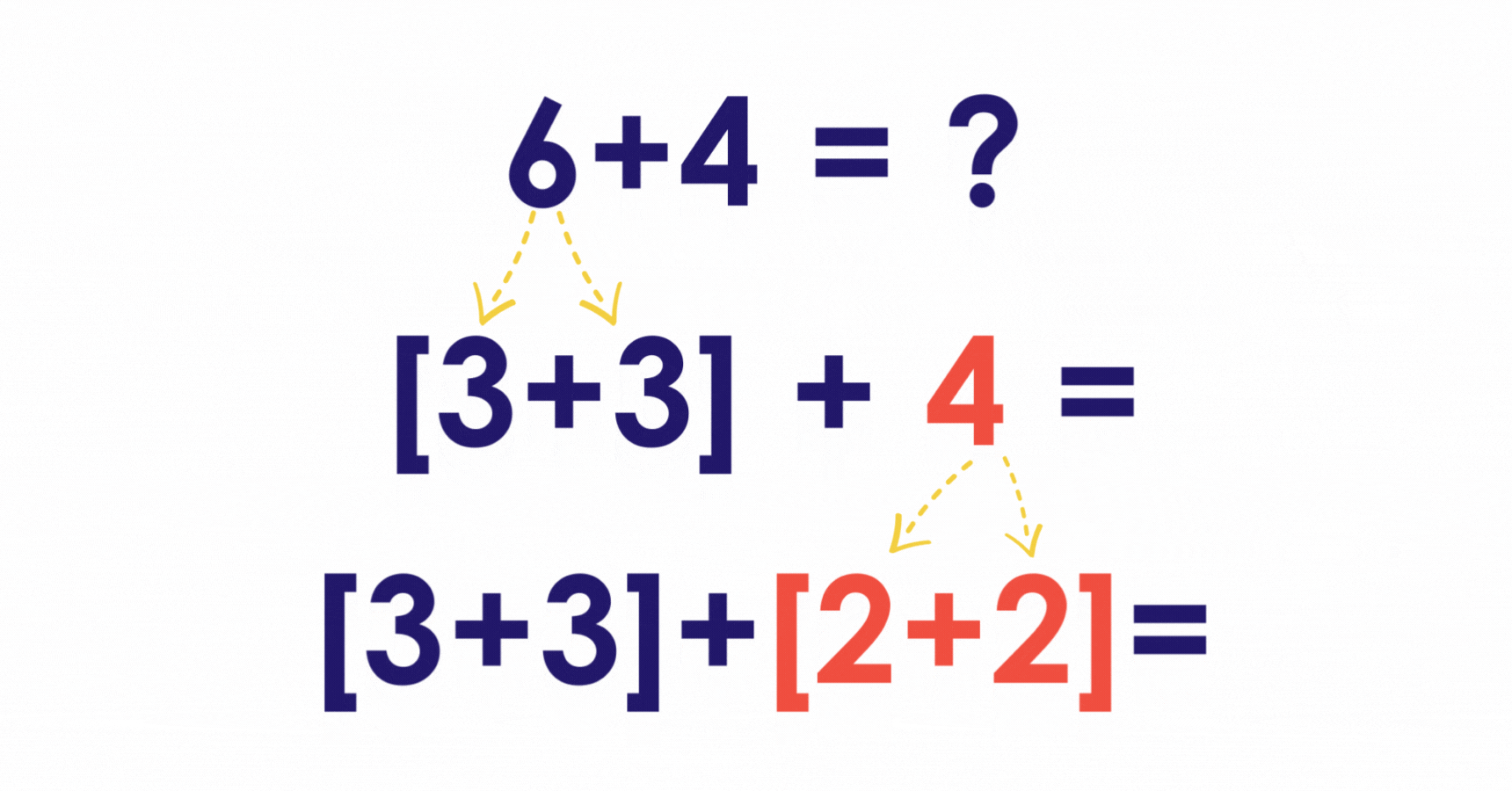
There are various strategies that rely on decomposition for larger sums, including

DIRECT RETRIEVAL
Students have stored the math fact in long-term memory and can retrieve it without falling back on a strategy.